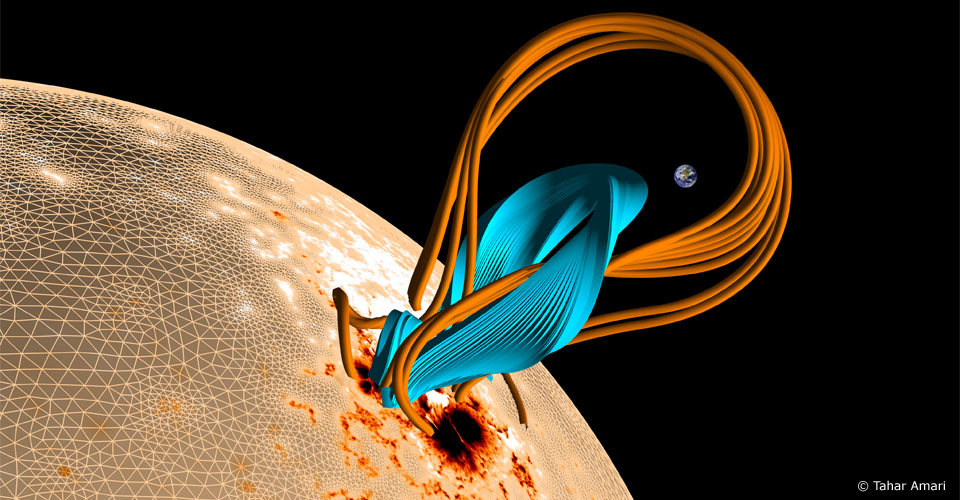
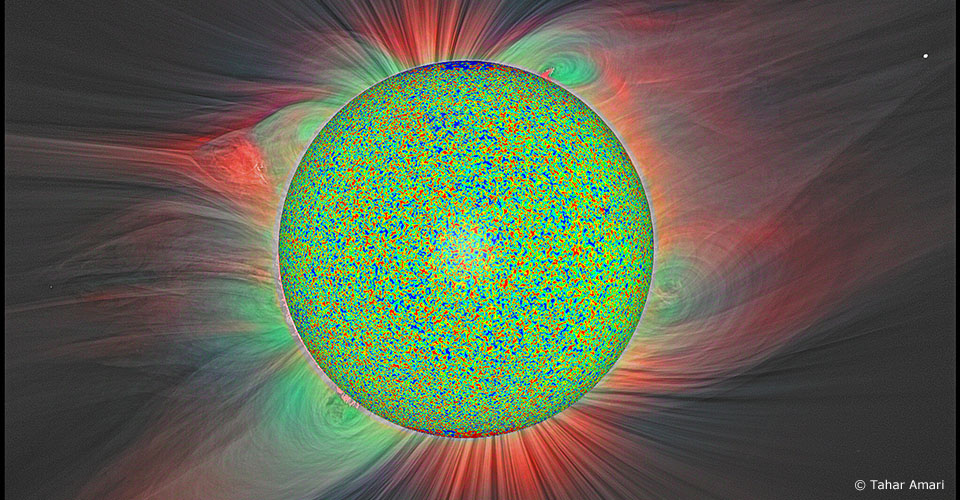
Le Centre de Physique Théorique (CPHT) réunit des chercheurs dont les activités couvrent un large spectre de la Physique, tant dans ses aspects fondamentaux qu'appliqués.
Le CPHT est une unité mixte de recherche (UMR 7644) du Centre National de la Recherche Scientifique (CNRS) et de l’Ecole polytechnique. Au niveau du CNRS, il est rattaché à l’Institut de physique. Le CPHT a également un partenariat avec le Collège de France.
Le CPHT, dirigé par Jean-René Chazottes, directeur de Recherche au CNRS, est implanté sur le campus de l’Ecole Polytechnique à Palaiseau, dans le bâtiment 6 et dans l'aile 0 du bâtiment 5. Le secrétariat se situe dans le Bâtiment 6, bureaux 06.1046 et 06.1045.
Adresse postale :
CPHT
Ecole Polytechnique
91128 Palaiseau cedex
France
Tél. Secrétariat : 01 69 33 42 01
Pour écrire un email à un membre du laboratoire : prenom.nom@polytechnique.edu